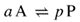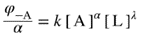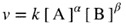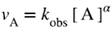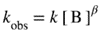| If the macroscopic (observed, empirical or phenomenological) rate of reaction (v) for any reaction can be expressed by an empirical differential rate equation (or rate law) which contains a factor of the form k [A]α [B]β ... (expressing in full the dependence of the rate of reaction on the concentrations [A], [B] ...) where α, β are constant exponents (independent of concentration and time) and k is independent of [A] and [B] etc. (rate constant, rate coefficient), then the reaction is said to be of order α with respect to A, of order β with respect to B, ... , and of (total or overall) order n = α + β + … The exponents α , β , ... can be positive or negative integral or rational nonintegral numbers. They are the reaction orders with respect to A, B, ... and are sometimes called ‘partial orders of reaction’. Orders of reaction deduced from the dependence of initial rates of reaction on concentration are called ‘orders of reaction with respect to concentration’; orders of reaction deduced from the dependence of the rate of reaction on time of reaction are called ‘orders of reaction with respect to time’. The concept of order of reaction is also applicable to chemical rate processes occurring in systems for which concentration changes (and hence the rate of reaction) are not themselves measurable, provided it is possible to measure a chemical flux. For example, if there is a dynamic equilibrium according to the equation:
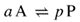
and if a chemical flux is experimentally found, (e.g. by NMR line-shape analysis) to be related to concentrations by the equation:
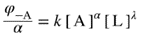
then the corresponding reaction is of order α with respect to A ... and of total (or overall) order n( = α + λ + ...). The proportionality factor k above is called the (nth order) ‘rate coefficient’. Rate coefficients referring to (or believed to refer to) elementary reactions are called ‘rate constants’ or, more appropriately ‘microscopic’ (hypothetical, mechanistic) rate constants. The (overall) order of a reaction cannot be deduced from measurements of a ‘rate of appearance’ or ‘rate of disappearance’ at a single value of the concentration of a species whose concentration is constant (or effectively constant) during the course of the reaction. If the overall rate of reaction is, for example, given by:
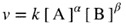
but [B] stays constant, then the order of the reaction (with respect to time), as observed from the concentration change of A with time, will be α, and the rate of disappearance of A can be expressed in the form:
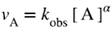
The proportionality factor kobs deduced from such an experiment is called the ‘observed rate coefficient’ and it is related to the (α + β)th order rate coefficient k by the equation:
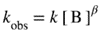
For the common case when α = 1, kobs is often referred to as a ‘pseudo-first order rate coefficient’ (kψ). For a simple (elementary) reactions a partial order of reaction is the same as the stoichiometric number of the reactant concerned and must therefore be a positive integer (see rate of reaction ). The overall order is then the same as the molecularity . For stepwise reactions there is no general connection between stoichiometric numbers and partial orders. Such reactions may have more complex rate laws, so that an apparent order of reaction may vary with the concentrations of the chemical species involved and with the progress of the reaction: in such cases it is not useful to speak of orders of reaction, although apparent orders of reaction may be deducible from initial rates. In a stepwise reaction, orders of reaction may in principle always be assigned to the elementary steps. |
თუ რეაქციის მაკროსკოპული (დაკვირვებული, ემპირიული ან ფენომენოლოგიური) სიჩქარე (v) ნებისმიერი რეაქციისთვის შეიძლება გამოისახოს ემპირიული დიფერენციალური სიჩქარის განტოლებით (ან სიჩქარის კანონით), რომელიც შეიცავს შესაბამის კოეფიციენტს k [A]α [B]β ... (სრულად გამოხატავს რეაქციის სიჩქარის დამოკიდებულებას [A], [B] ... კონცენტრაციებზე), სადაც α, β მუდმივი ხარისხის მაჩვენებლებია (არაა დამოკიდებული კონცენტრაციასა და დროზე), ხოლო k არ არის დამოკიდებული [A]-ზე, [B]-სა და ა. შ. (სიჩქარის მუდმივა, სიჩქარის კოეფიციენტი), მაშინ ამბობენ, რომ რეაქცია არის α რიგის A-ს მიმართ, β რიგის B-ს მიმართ და ა. შ., და ჯამური n რიგის (n = α + β + …). α , β , ... შეიძლება იყოს დადებითი ან უარყოფითი მთელი ან რაციონალური არამთელი რიცხვები. ისინი წარმოადგენს რეაქციის რიგს A, B, ... მიმართ და ზოგჯერ იწოდება როგორც ″რეაქციის ნაწილობრივი რიგი″. რეაქციის რიგს, რომელიც გამოიყვანება რეაქციის საწყისი სიჩქარის კონცენტრაციაზე დამოკიდებულებიდან, ეწოდება ″რეაქციის რიგი კონცენტრაციის მიხედვით″; რეაქციის რიგს, რომელიც გამოიყვანება რეაქციის სიჩქარის რეაქციის დროზე დამოკიდებულებიდან, ეწოდება ″რეაქციის რიგი დროის მიმართ″. რეაქციის რიგის კონცეფცია ასევე გამოიყენება ქიმიური პროცესებისათვის, რომლებიც მიმდინარეობს სისტემებში, რომლებშიც კონცენტრაციის ცვლილება (და, შესაბამისად, რეაქციის სიჩქარე) თავისთავად არ არის გაზომვადი, იმ პირობით, რომ შესაძლებელია ქიმიური ნაკადის გაზომვა. მაგ., თუ არსებობს დინამიკური წონასწორობა:
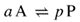
და თუ ექსპერიმენტულად (მაგ., ბმრ სიგნალის ფორმის ანალიზით) დადგენილია, რომ ქიმიური ნაკადი დაკავშირებულია კონცენტრაციებთან განტოლებით:
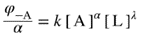
მაშინ შესაბამისი რეაქცია არის α რიგის A-ს მიმართ და ... საერთო (ან ჯამური) რიგია n( = α + λ + ...). ზევით მოყვანილი პროპორციულობის კოეფიციენტს (k) ეწოდება (მე-n რიგის) „სიჩქარის კოეფიციენტი″. სიჩქარის კოეფიციენტებს, რომლებიც მიეკუთვნება (ან სავარაუდოდ მიეკუთვნება) ელემენტარულ რეაქციებს, ეწოდება ″სიჩქარის მუდმივები″ ან, უფრო სწორად, ″მიკროსკოპული″ (ჰიპოთეტური, მექანისტური) სიჩქარის მუდმივები. რეაქციის (საერთო) რიგის დადგენა შეუძლებელია „წარმოქმნის სიჩქარის″ ან „ხარჯვის სიჩქარის″ გაზომვით იმ ნაწილაკის კონცენტრაციის ერთი მნიშვნელობით, რომლის კონცენტრაცია მუდმივია (ან ფაქტობრივად მუდმივია) რეაქციის მიმდინარეობისას. თუ რეაქციის საერთო სიჩქარე განისაზღვრება, მაგ., ფორმულით:
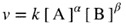
მაგრამ [B] რჩება მუდმივი, მაშინ რეაქციის რიგი (დროსთან მიმართებაში), როგორც ეს შეინიშნება A-ს კონცენტრაციის დროში ცვლილებიდან, იქნება α, ხოლო A-ს ხარჯვის სიჩქარე შეიძლება გამოიხატოს შემდეგი სახით:
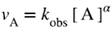
პროპორციულობის კოეფიციენტს kobs, რომელიც გამოყვანილია ასეთი ექსპერიმენტიდან, ეწოდება ″დაკვირვებული სიჩქარის კოეფიციენტი″ და იგი დაკავშირებულია (α + β)-ე რიგის სიჩქარის k კოეფიციენტთან შემდეგი განტოლებით:
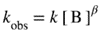
ზოგადი შემთხვევისთვის, როდესაც α = 1, kobs-ს ხშირად უწოდებენ ″ფსევდო-პირველი რიგის სიჩქარის კოეფიციენტს″ (kψ). მარტივი (ელემენტარული) რეაქციებისთვის, რეაქციის ნაწილობრივი რიგი ემთხვევა შესაბამისი რეაგენტის სტექიომეტრიულ რიცხვს და, შესაბამისად, უნდა იყოს დადებითი მთელი რიცხვი (იხ. რეაქციის სიჩქარე). მაშინ საერთო რიგი იგივეა, რაც მოლეკულურობა. საფეხურებრივი რეაქციებისთვის არ არსებობს ზოგადი კავშირი სტექიომეტრიულ რიცხვებსა და ნაწილობრივ რიგებს შორის. ასეთ რეაქციებისთვის სიჩქარის კანონები უფრო რთულია, ასე რომ, რეაქციის მოჩვენებითი რიგი შეიძლება განსხვავდებოდეს მონაწილე ქიმიური ნაწილაკების კონცენტრაციისა და რეაქციის მიმდინარეობის მიხედვით: ასეთ შემთხვევებში რეაქციის რიგების განხილვა არ არის მიზანშეწონილი. რეაქციის რიგი შეიძლება გამოყვანილ იქნეს საწყისი სიჩქარიდან. საფეხურებრივი რეაქციის დროს, რეაქციის რიგები, პრინციპში, ყოველთვის შეიძლება მიეკუთვნებოდეს ელემენტარულ ეტაპებს. |